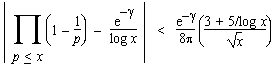Mertens' theorem
(another Prime Pages' Glossary entries)
The Prime Glossary
Glossary: Prime Pages: Top 5000: Mertens used Chebyshev's theorem (a weak version of the prime number theorem) to prove that
.
This is now called Mertens' Theorem.

Assuming the Riemann hypothesis, Schoenfeld showed that when x > 8, we have the following error bound

where is Euler's constant.

Related pages (outside of this work)

References:

BS96 (p. 210,234)
E. Bach and J. Shallit, Algorithmic number theory, Foundations of Computing volume I: Efficient Algorithms, The MIT Press, Cambridge, MA, pp. xvi+512, 1996.  MR 97e:11157 (Annotation available)


Chris Caldwell © 1999-2010 (all rights reserved)